In Sri Lanka, university admissions are determined through a standardized method known as the Z-score, which ensures a fair comparison of student performance across various subjects in the GCE Advanced Level examinations. This approach addresses disparities in subject difficulty and marking schemes, facilitating an equitable selection process for higher education.
Understanding the Z-Score Calculation
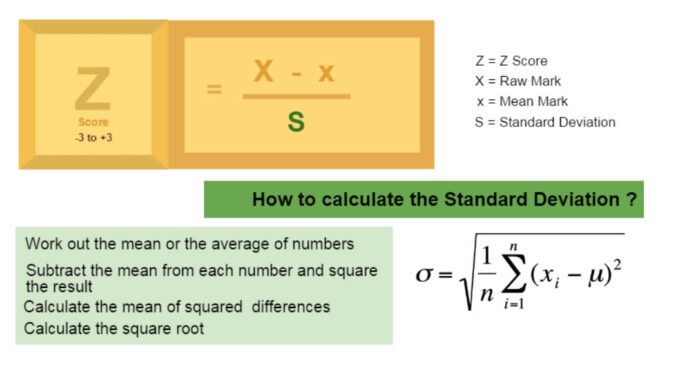
The Z-score represents how a student’s performance in a particular subject deviates from the average performance of all candidates in that subject. It’s calculated using the formula:
Z = (X – μ) / σ
Where:
- X is the student’s raw mark in the subject.
- μ is the mean mark of all candidates in that subject.
- σ is the standard deviation of marks in that subject.
This calculation standardizes the scores, producing a mean of zero and a standard deviation of one for each subject. Consequently, Z-scores typically range from approximately -3 to +3. A positive Z-score indicates a performance above the mean, while a negative score signifies below-average performance.
Application in University Admissions
For university selection, a candidate’s Z-scores across their subjects are averaged to produce a composite Z-score. This composite score enables a fair ranking of students, irrespective of the subjects they have chosen. By standardizing scores, the system mitigates the impact of varying subject difficulties and marking stringencies, ensuring that students are assessed on a level playing field.
District Quota System
Sri Lanka employs a district quota system to promote equitable access to university education across different regions. University admissions are allocated as follows:
- 40% on an all-island merit basis.
- 55% based on district quotas, proportionate to the population of each district.
- 5% reserved for educationally disadvantaged districts, also allocated based on population.
The University Grants Commission has identified 13 educationally disadvantaged districts, including Nuwara-Eliya, Hambantota, Jaffna, and others. This quota system ensures that students from all regions, especially those from underprivileged areas, have fair opportunities for university admission.
Conclusion
The Z-score system in Sri Lanka plays a pivotal role in maintaining fairness and transparency in university admissions. By standardizing student performance across diverse subjects and incorporating regional quotas, the system strives to provide equitable access to higher education for students nationwide.
For a more detailed explanation and examples of Z-score calculations, you can refer to the original article on Student Lanka:
What is the Z-Score?
The Z-score is a statistical measure used to indicate how a specific data point relates to the mean of a dataset. In the context of education, such as university selection in Sri Lanka, it shows how a student’s performance compares to the average performance of all students in a particular subject.
The Z-score formula standardizes individual scores, making it possible to compare performances across different subjects and exams that may have varying levels of difficulty or marking schemes.
How is the Z-Score Calculated?
The Z-score formula is:
Z=X−μσZ = \frac{X – \mu}{\sigma}Z=σX−μ
Where:
- X: The raw score (student’s marks in the subject).
- μ (mu): The mean (average) of all students’ scores in the subject.
- σ (sigma): The standard deviation of scores in the subject, which measures the spread of marks around the mean.
Steps to Calculate Z-Score:
- Find the Mean (μ):
- Add up all the marks scored by students in a subject and divide by the total number of students.
- Calculate the Standard Deviation (σ):
- Determine the deviation of each mark from the mean, square these deviations, average them, and then take the square root.
- Apply the Formula:
- Substitute the student’s mark, mean, and standard deviation into the formula to calculate the Z-score.
Example:
Consider two students, A and B, in the same subject:
- Marks scored by all students: 50, 55, 60, 70, 75
- Student A scored: 60
- Student B scored: 75
Step 1: Find the Mean
μ=50+55+60+70+755=62\mu = \frac{50 + 55 + 60 + 70 + 75}{5} = 62μ=550+55+60+70+75=62
Step 2: Calculate the Standard Deviation
- Calculate the deviations:
- (50-62)² = 144
- (55-62)² = 49
- (60-62)² = 4
- (70-62)² = 64
- (75-62)² = 169
- Average of deviations:
Variance=144+49+4+64+1695=86\text{Variance} = \frac{144 + 49 + 4 + 64 + 169}{5} = 86Variance=5144+49+4+64+169=86 - Standard deviation:
σ=86≈9.27\sigma = \sqrt{86} \approx 9.27σ=86≈9.27
Step 3: Calculate Z-scores for A and B
- Student A:
ZA=60−629.27≈−0.22Z_A = \frac{60 – 62}{9.27} \approx -0.22ZA=9.2760−62≈−0.22 - Student B:
ZB=75−629.27≈1.40Z_B = \frac{75 – 62}{9.27} \approx 1.40ZB=9.2775−62≈1.40
Comparing the Z-Scores
- Student A’s Z-score (-0.22): Slightly below the average.
- Student B’s Z-score (1.40): Well above the average.
Conclusion:
Student B performed significantly better than Student A in terms of deviation from the average score. The Z-score allows for a precise comparison of their relative performance, accounting for the overall performance distribution.
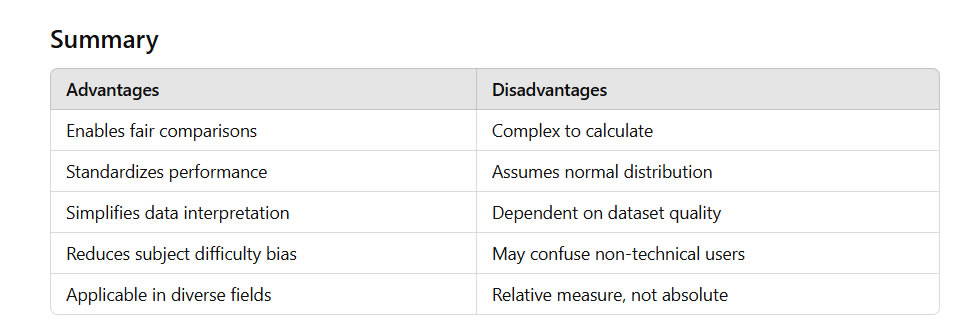
Benefits and Disadvantages of the Z-Score
Benefits of Z-Score
- Standardization of Scores
- Z-scores allow comparisons across different subjects, exams, or datasets by normalizing scores to a common scale, regardless of the original range or difficulty.
- Fairness in Comparison
- Especially in education, Z-scores ensure that variations in subject difficulty and marking are accounted for, providing a level playing field for all students.
- Simplified Interpretation
- A Z-score indicates how far a value is from the mean in terms of standard deviations, making it easy to identify above-average, average, or below-average performances.
- Useful in Selection Processes
- In university admissions, Z-scores help rank students fairly, combining performance from multiple subjects into a single standardized metric.
- Wide Applicability
- Z-scores are widely used in fields like statistics, finance, psychology, and education for measuring relative performance or identifying outliers.
- Handles Diverse Data Distributions
- Z-scores accommodate datasets with varying spreads, as they use the standard deviation to adjust for differences in variability.
Disadvantages of Z-Score
- Complex Calculations
- Calculating Z-scores involves determining the mean and standard deviation, which can be time-consuming and prone to errors, especially with large datasets.
- Dependence on Dataset Quality
- If the dataset contains errors, outliers, or is not representative of the population, the Z-score results may be misleading.
- Not Intuitive for Everyone
- While Z-scores are mathematically meaningful, they may be confusing for those unfamiliar with standard deviations and statistical concepts.
- Assumes Normal Distribution
- Z-scores are most effective when the data is normally distributed. If the dataset deviates significantly from normality, the interpretation of Z-scores becomes less accurate.
- Relative, Not Absolute
- Z-scores measure performance relative to others in the dataset, not an absolute performance level. For example, a high Z-score in an easy subject may not reflect true capability.
- Subject Bias in Educational Contexts
- In cases where subject choices are vastly different in terms of popularity or complexity, even standardized scores might introduce biases in comparative rankings.
Conclusion:
While the Z-score is a powerful tool for normalization and comparison, it must be used thoughtfully, ensuring the dataset’s accuracy and relevance. For critical decisions like university admissions, its strengths often outweigh its limitations.
Refer 2023(2024) Minimum “Z” Scores for selection to various Courses


Leave a Reply
You must be logged in to post a comment.